- Mixed In Key Coupon, Navisworks Freedom 2011 Free Download, Omni Group OmniGraffle 5 Standard Serial Key, Infinite Skills - Learning FileMaker Pro 12 Iso Online Tools Business and Productivity 14 Image Design or Photo 3 Utility 1 Video 1.
- Calculate out how many times the denominator goes into the numerator. To do that, divide 35 by 10 and keep only what is to the left of the decimal point: 35 / 10 = 3.5000 = 3. Step 2 - Find New Numerator. Multiply the answer from Step 1 by the denominator and deduct that from the original numerator. 35 - ( 10 x 3) = 5. Step 3 - Put Together.
Striking the F4 key multiple times helps in changing the position of the dollar sign. It is also to be noted that mixed reference cannot be pasted into a Table. We can only create an absolute or relative reference in a table. We can use the excel shortcut ALT+36 or Shift+4 key for inserting the dollar sign in Excel.
Introduction to Fractions
In previous class we learnt following things about fractions.
a) Meaning of Fractions
b) Meaning of Numerator and denominator
c) Equivalent Fractions
d) Comparison of fractions
e) Addition of fractions
f) Subtraction of fractions
A fraction represents a part of the whole. For example, 1⁄2 is a fraction, that means 1 part out of 2 equal parts.
1⁄4 is a fraction, it denotes 1 part out of 4 equal parts.
3⁄8 is a fraction, it denotes 3 equal parts out of 8 equal parts. Here 3 is numerator and 8 is denominator.
Type of Fractions
There are 4 types of fractions and they are mentioned below.
a) Unit Fractions
b) Proper fractions
c) Improper fractions
d) Mixed fractions
Unit Fractions
Fractions which have 1 in their numerator are known as unit fractions. Few examples are given below.
1⁄2, 1⁄3, 1⁄4, 1⁄5, 1⁄9, 1⁄21, etc.
Proper Fractions
Fractions whose numerator is smaller than the denominator are known as proper fractions. Few examples are given below.
2⁄5, 5⁄7, 3⁄7, 7⁄12, etc.
Improper Fractions
Fractions whose numerator is greater than or equal to the denominator are known as improper fractions. Few examples are given below.
5⁄2, 7⁄4, 25⁄27, 43⁄21, etc.

Mixed Fractions
This type of fractions are combination of a whole number and a proper fraction. Few examples are given below.
11⁄2, 22⁄3, 35⁄7, 51⁄5, etc.
Conversion of Fractions
We can convert improper fractions to mixed fractions and vice versa. Let's have a look at some examples provided below.
Conversion of Improper Fractions to Mixed Fractions
Improper fractions can be converted into mixed fractions by dividing the numerator by the denominator. The quotient gives the whole number part of the mixed fractions, and the remainder placed over the denominator gives the fractional part.
Example 1. Convert 10⁄3 into a mixed fraction.
Solution.
So, the answer will be 31⁄3.
Example 2. Convert 112⁄20 into a mixed fraction.
Solution.
Quotient 5 will be treated as whole number of mixed fraction.
Fraction part of the mixed fraction = 12⁄20 = 3⁄5
So, the mixed fraction will be 53⁄5.
Conversion of Mixed Fractions into Improper Fractions
A mixed fraction can be changed into an improper fraction by multiplying the whole number by the denominator of the fraction and adding the product to numerator of the fraction.
Comparison of Fractions
Comparison of Like Fractions
If both the fractions denominators are same, then we compare the numerators of both the fractions. The fraction which has bigger numerator is the bigger fraction.
Example 1. Which of the fraction is smaller 3⁄5 or 2⁄5 ?
Solution. As both the fractions have same denominator, we must compare the numerators.
3 > 2. So, 3⁄5 >2⁄5.
Example 2. Which of the fraction is smaller 106⁄11 or 123⁄11 ?
Solution. Both fractions numerator are same, that is 11. Now we need to compare the numerators.
123 > 106. So, 123⁄11 >106⁄11.
Comparison of Unlike Fraction with Same Numerator
When the numerator of both the fractions are same, then the fraction which is having smaller denominator is the bigger fraction.
Example 1. Which of the following fraction is greater, 25⁄7 or 25⁄17 ?
Solution. In this comparison both the fractions are having same numerator that is 25. Now, we need to compare the denominators of both the fractions.
Here 7 < 17, that means 25⁄7 >25⁄17.
Example 2. Which of the following fraction is greater, 105⁄109 or 105⁄89 ?
Solution. Both the fractions are having same numerator that is 105. Now, time to compare the denominators of both the fractions, 89 > 109.
So, 105⁄89 >105⁄109.
Comparison of Unlike Fractions with Different Numerator and Different Denominator
When both the numerator and denominator of both fractions are different, then to find greater fraction we need to do following things. Let's consider 3⁄7 and 2⁄5 for comparison.
Step-1
we multiply 1st fraction's denominator with both the numerator and denominator of the 2nd fraction. 1st fraction denominator is 7, we have to multiply it to both numerator and denominator of 2⁄5.
Step-2
Similarly, we need to multiply 2nd fraction's denominator 5 with both numerator and denominator 3⁄7.
Step-3
Now comparing 14⁄35 and 15⁄35 became easy as both fractions denominator became same. So, 14⁄35< 15⁄35 or
Comparison of Mixed Fractions
For comparing mixed fractions, we have to check the whole numbers first, if the whole numbers are same then we have to compare the fractions.
Example 1. Compare 52⁄5 and 41⁄3.
Solution. Whole number of 52⁄5 is 5 and the whole number of 41⁄3 is 4.
5 > 4. So, 52⁄5 > 41⁄3.
Example 2. Compare 12⁄3 and 12⁄5.
Solution. Whole number of both the fractions are same here. We can not decide which fraction is bigger considering the whole number. Now, we need to check the fraction part, 2⁄3 and 2⁄5. Among these two fractions, 2⁄3 is bigger than 2⁄5.
So, 12⁄3 > 12⁄5.
Addition of Fractions
In our previous class we learnt the basics of fraction addition. Here we will learn various types of fraction addition.
Addition of Fractions with Same Denominator
When both the fractions have same denominator, we first add the numerators and then place the sum over the common denominator. We simplify further if needed.
Please have a look at the below given video for better understanding.
Addition of Fractions with different Denominators
In this kind of addition, we first find the LCM of all the denominators. Then convert all the fractions into equivalent fractions having common denominators as LCM. Then we add all the equivalent fractions. Let's have a look at some examples.
Please have a look at the below given video for better understanding.
Example 1. Add 3⁄8 and 2⁄6.
Solution. LCM of 8 and 6 is 24.
To get the equivalent fraction of 3⁄8 whose denominator should be 24, we need to do the following.
Similarly, to get the equivalent fraction of 2⁄6 whose denominator should be 24, we need to do the following.
Add both the equivalent fractions.
So, the answer is 17⁄24.
Addition of Mixed Fractions
First convert the mixed fractions into improper fractions and then add them as normal fractions rule. Let's have a look at some examples.
Example 1. Add 23⁄5 and 32⁄3.
Solution. 23⁄5 is equal to 13⁄5.
32⁄3 is equal to 11⁄3.
Now, we have to follow the addition procedure by taking out the LCM of the denominators 5 and 3.
LCM of 5 and 3 = 15
This can be done in another method.
First add the whole numbers, that is, 2 + 3 = 5 and keep it on the left side.
Now add the fractions 3⁄5 and 2⁄3 =
Now, we have to follow the addition procedure by taking out the LCM of the denominators 5 and 3.
LCM of 5 and 3 = 15
We can further simplify 19/15 to mixed fraction = 19⁄15 = 14⁄15
We have whole numbers 5 which came from earlier whole numbers addition.
So, the total sum =
Please visit below mentioned links for more fraction addition examples and worksheet.
Fraction AdditionSubtraction of Fractions
In our previous class we learnt the basics of fraction subtraction. Here we will learn various types of fraction subtraction.
Winclone 6 2 – clone your boot camp partition usb. Subtraction of Fractions with Equal Denominator
To subtract fractions having equal denominator, we first subtract the numerators. Then we have to place the answer over the denominator. Let's have a look at some examples.
Please have a look at the below given video for better understanding.
Example 1. Subtract 2⁄5 from 4⁄5.
Solution.
Example 2. Subtract 25⁄7 from 35⁄7.
Solution.
Subtraction of Fractions with Different Denominators
To subtract fractions having different denominators, we first take out the LCM of the denominators. Then convert all the fractions into equivalent fractions having common denominators as LCM. Then we subtract equivalent fractions. Let's have a look at some examples.
Example 1. Subtract 3⁄4 from 7⁄8.
Solution. LCM of 4 and 8 is 8.
Example 2. Subtract 5⁄25 from 3⁄5.
Solution. LCM of 5 and 25 is 25.
10⁄25 can be simplified further, that is, 2⁄5.
So, 2⁄5 is the answer.
Please have a look at the below given video for better understanding.
Subtraction of Mixed Fractions
In this kind of subtraction, first convert the mixed fractions into improper fractions and then subtract them as normal fractions rule. Let's have a look at some examples.
Example 1. Subtract 22⁄5 from 32⁄3.
Solution. Improper fraction of 22⁄5 =
Improper fraction of 32⁄3 =
LCM of 3 and 5 is 15
So, the answer is 14⁄15.
Subtraction from a Whole number
When we subtract a fraction from a whole number, we must convert the whole number to its fraction format. Then do the subtraction as per normal rules we learnt till now.
Example 1. Subtract 6⁄7 from 2.
Solution. Fraction format of 2 is 2⁄1.Now, subtract 6⁄7 from 2⁄1.
So, the answer is 11⁄7.
Multiplication of Fractions
Multiplication of Fraction by a Whole Number
To multiply a fraction by a whole number, we multiply the numerator of the fraction by the whole number and denominator of the fraction by 1. Let's have a look at some examples.
Example 1. Multiply 4⁄7 by 5.
Solution.
So, the answer is 26⁄7.
Multiplication of Two Fractions
To multiply two fractions, we multiply the numerators of both the fractions and the product becomes numerator. Then, we multiply denominators of both the fractions and the product becomes denominator of the result. Let's have a look at some examples.
Example 1. Multiply 4⁄5 by 1⁄2.
Solution.
If we simplify 4⁄10 further, then it will become 2⁄5.
So, the answer is 2⁄5.
Example 2. Multiply 22⁄5 by 32⁄3.
Solution. Here, we have two mixed fractions. First, we must convert them to improper fractions, then we do the multiplication.
Improper fraction of 22⁄5 = 12⁄5
Improper fraction of 32⁄3 = 11⁄3
Now multiply
So, the answer is 812⁄15.
Please have a look at the below given video for better understanding.
Reciprocal of Fractions
Two fractions whose product is 1 are called reciprocals. For example, 2⁄3 reciprocal is 3⁄2. If the product of two fractions is equal to 1 then we can say 2⁄3 and 3⁄2 are reciprocals.
In other words, if we want to find out the reciprocal of a fraction then interchange its numerator to denominator and
denominator to numerator.
Example 1. What is the reciprocal of 5⁄7?
Solution. Reciprocal of 5⁄7 is 7⁄5.
Division of Fractions
In the division of fraction, we must find out the reciprocal of divisor and multiply it with the dividend. Let's have a look at some example.
Example 1. Divide 5⁄8 by 1⁄2.
Solution.5⁄8 ÷ 1⁄2
Here, 5⁄8 is the dividend and 1⁄2 is divisor. Reciprocal of 1⁄2 is 2⁄1.
So, the answer is 5⁄4.
Please have a look at the below given video for better understanding.
Fractions Test
Fractions Test - 1Fractions Test - 2Fractions Test - 3Class-4 Fractions Worksheet
Fractions Worksheet - 1Fractions Worksheet - 2Fractions Worksheet - 3Fractions Worksheet - 4Fractions Worksheet - 5Fractions Worksheet - 6Fractions Worksheet - 7Fractions Worksheet - 8Answer Sheet
Fractions-AnswerDownload the pdf
Copyright © 2021 LetsPlayMaths.com. All Rights Reserved.
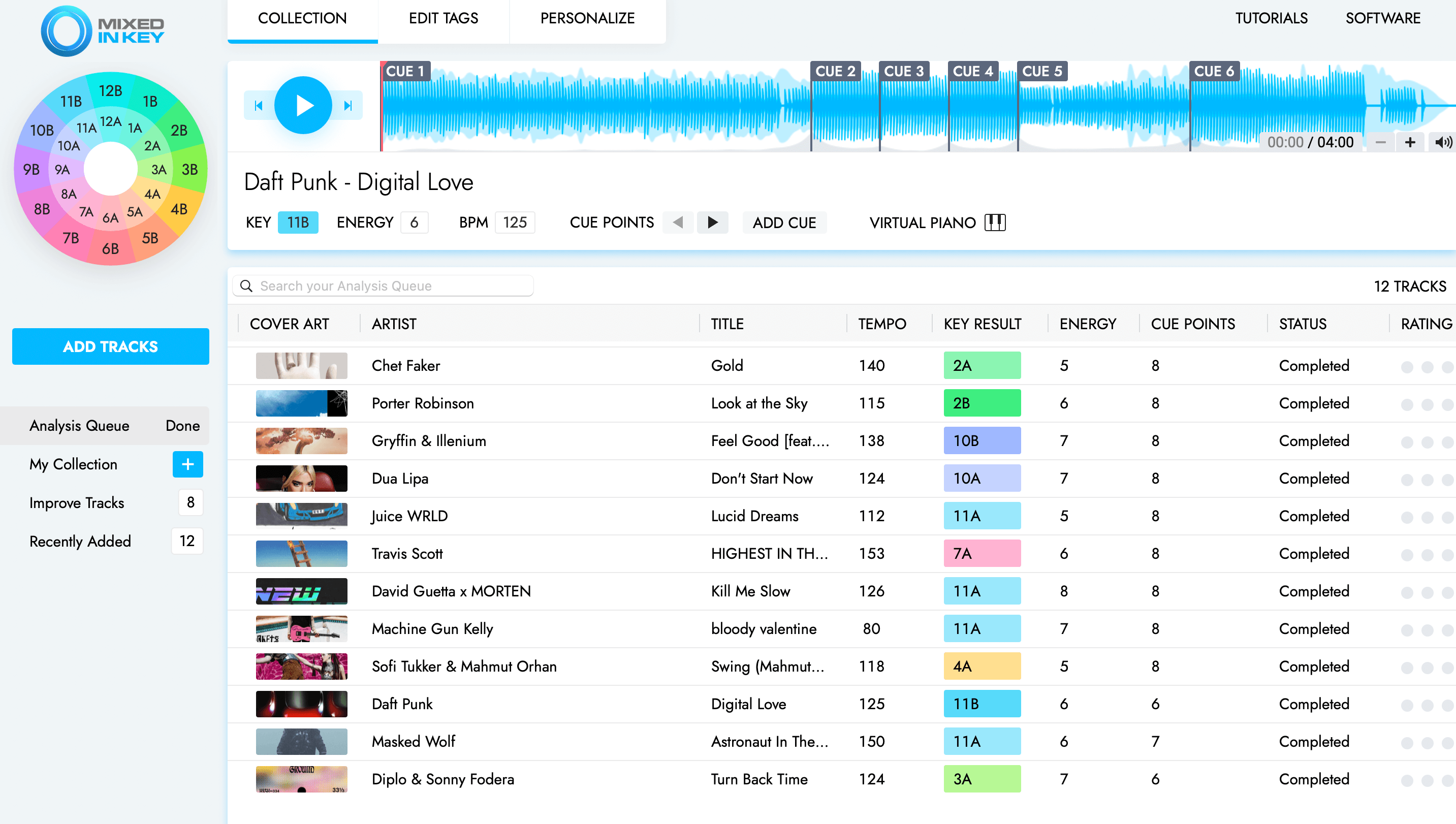
Mixed In Key 8 5 35 5 6
Below are multiple fraction calculators capable of addition, subtraction, multiplication, division, simplification, and conversion between fractions and decimals. Fields above the solid black line represent the numerator, while fields below represent the denominator.
Mixed Numbers Calculator
Simplify Fractions Calculator
Decimal to Fraction Calculator
Fraction to Decimal Calculator
Big Number Fraction Calculator
Use this calculator if the numerators or denominators are very big integers.
In mathematics, a fraction is a number that represents a part of a whole. It consists of a numerator and a denominator. The numerator represents the number of equal parts of a whole, while the denominator is the total number of parts that make up said whole. For example, in the fraction of
| 3 |
| 8 |
| 5 |
| 8 |
Mixed Fractions
This type of fractions are combination of a whole number and a proper fraction. Few examples are given below.
11⁄2, 22⁄3, 35⁄7, 51⁄5, etc.
Conversion of Fractions
We can convert improper fractions to mixed fractions and vice versa. Let's have a look at some examples provided below.
Conversion of Improper Fractions to Mixed Fractions
Improper fractions can be converted into mixed fractions by dividing the numerator by the denominator. The quotient gives the whole number part of the mixed fractions, and the remainder placed over the denominator gives the fractional part.
Example 1. Convert 10⁄3 into a mixed fraction.
Solution.
So, the answer will be 31⁄3.
Example 2. Convert 112⁄20 into a mixed fraction.
Solution.
Quotient 5 will be treated as whole number of mixed fraction.
Fraction part of the mixed fraction = 12⁄20 = 3⁄5
So, the mixed fraction will be 53⁄5.
Conversion of Mixed Fractions into Improper Fractions
A mixed fraction can be changed into an improper fraction by multiplying the whole number by the denominator of the fraction and adding the product to numerator of the fraction.
Comparison of Fractions
Comparison of Like Fractions
If both the fractions denominators are same, then we compare the numerators of both the fractions. The fraction which has bigger numerator is the bigger fraction.
Example 1. Which of the fraction is smaller 3⁄5 or 2⁄5 ?
Solution. As both the fractions have same denominator, we must compare the numerators.
3 > 2. So, 3⁄5 >2⁄5.
Example 2. Which of the fraction is smaller 106⁄11 or 123⁄11 ?
Solution. Both fractions numerator are same, that is 11. Now we need to compare the numerators.
123 > 106. So, 123⁄11 >106⁄11.
Comparison of Unlike Fraction with Same Numerator
When the numerator of both the fractions are same, then the fraction which is having smaller denominator is the bigger fraction.
Example 1. Which of the following fraction is greater, 25⁄7 or 25⁄17 ?
Solution. In this comparison both the fractions are having same numerator that is 25. Now, we need to compare the denominators of both the fractions.
Here 7 < 17, that means 25⁄7 >25⁄17.
Example 2. Which of the following fraction is greater, 105⁄109 or 105⁄89 ?
Solution. Both the fractions are having same numerator that is 105. Now, time to compare the denominators of both the fractions, 89 > 109.
So, 105⁄89 >105⁄109.
Comparison of Unlike Fractions with Different Numerator and Different Denominator
When both the numerator and denominator of both fractions are different, then to find greater fraction we need to do following things. Let's consider 3⁄7 and 2⁄5 for comparison.
Step-1
we multiply 1st fraction's denominator with both the numerator and denominator of the 2nd fraction. 1st fraction denominator is 7, we have to multiply it to both numerator and denominator of 2⁄5.
Step-2
Similarly, we need to multiply 2nd fraction's denominator 5 with both numerator and denominator 3⁄7.
Step-3
Now comparing 14⁄35 and 15⁄35 became easy as both fractions denominator became same. So, 14⁄35< 15⁄35 or
Comparison of Mixed Fractions
For comparing mixed fractions, we have to check the whole numbers first, if the whole numbers are same then we have to compare the fractions.
Example 1. Compare 52⁄5 and 41⁄3.
Solution. Whole number of 52⁄5 is 5 and the whole number of 41⁄3 is 4.
5 > 4. So, 52⁄5 > 41⁄3.
Example 2. Compare 12⁄3 and 12⁄5.
Solution. Whole number of both the fractions are same here. We can not decide which fraction is bigger considering the whole number. Now, we need to check the fraction part, 2⁄3 and 2⁄5. Among these two fractions, 2⁄3 is bigger than 2⁄5.
So, 12⁄3 > 12⁄5.
Addition of Fractions
In our previous class we learnt the basics of fraction addition. Here we will learn various types of fraction addition.
Addition of Fractions with Same Denominator
When both the fractions have same denominator, we first add the numerators and then place the sum over the common denominator. We simplify further if needed.
Please have a look at the below given video for better understanding.
Addition of Fractions with different Denominators
In this kind of addition, we first find the LCM of all the denominators. Then convert all the fractions into equivalent fractions having common denominators as LCM. Then we add all the equivalent fractions. Let's have a look at some examples.
Please have a look at the below given video for better understanding.
Example 1. Add 3⁄8 and 2⁄6.
Solution. LCM of 8 and 6 is 24.
To get the equivalent fraction of 3⁄8 whose denominator should be 24, we need to do the following.
Similarly, to get the equivalent fraction of 2⁄6 whose denominator should be 24, we need to do the following.
Add both the equivalent fractions.
So, the answer is 17⁄24.
Addition of Mixed Fractions
First convert the mixed fractions into improper fractions and then add them as normal fractions rule. Let's have a look at some examples.
Example 1. Add 23⁄5 and 32⁄3.
Solution. 23⁄5 is equal to 13⁄5.
32⁄3 is equal to 11⁄3.
Now, we have to follow the addition procedure by taking out the LCM of the denominators 5 and 3.
LCM of 5 and 3 = 15
This can be done in another method.
First add the whole numbers, that is, 2 + 3 = 5 and keep it on the left side.
Now add the fractions 3⁄5 and 2⁄3 =
Now, we have to follow the addition procedure by taking out the LCM of the denominators 5 and 3.
LCM of 5 and 3 = 15
We can further simplify 19/15 to mixed fraction = 19⁄15 = 14⁄15
We have whole numbers 5 which came from earlier whole numbers addition.
So, the total sum =
Please visit below mentioned links for more fraction addition examples and worksheet.
Fraction AdditionSubtraction of Fractions
In our previous class we learnt the basics of fraction subtraction. Here we will learn various types of fraction subtraction.
Winclone 6 2 – clone your boot camp partition usb. Subtraction of Fractions with Equal Denominator
To subtract fractions having equal denominator, we first subtract the numerators. Then we have to place the answer over the denominator. Let's have a look at some examples.
Please have a look at the below given video for better understanding.
Example 1. Subtract 2⁄5 from 4⁄5.
Solution.
Example 2. Subtract 25⁄7 from 35⁄7.
Solution.
Subtraction of Fractions with Different Denominators
To subtract fractions having different denominators, we first take out the LCM of the denominators. Then convert all the fractions into equivalent fractions having common denominators as LCM. Then we subtract equivalent fractions. Let's have a look at some examples.
Example 1. Subtract 3⁄4 from 7⁄8.
Solution. LCM of 4 and 8 is 8.
Example 2. Subtract 5⁄25 from 3⁄5.
Solution. LCM of 5 and 25 is 25.
10⁄25 can be simplified further, that is, 2⁄5.
So, 2⁄5 is the answer.
Please have a look at the below given video for better understanding.
Subtraction of Mixed Fractions
In this kind of subtraction, first convert the mixed fractions into improper fractions and then subtract them as normal fractions rule. Let's have a look at some examples.
Example 1. Subtract 22⁄5 from 32⁄3.
Solution. Improper fraction of 22⁄5 =
Improper fraction of 32⁄3 =
LCM of 3 and 5 is 15
So, the answer is 14⁄15.
Subtraction from a Whole number
When we subtract a fraction from a whole number, we must convert the whole number to its fraction format. Then do the subtraction as per normal rules we learnt till now.
Example 1. Subtract 6⁄7 from 2.
Solution. Fraction format of 2 is 2⁄1.Now, subtract 6⁄7 from 2⁄1.
So, the answer is 11⁄7.
Multiplication of Fractions
Multiplication of Fraction by a Whole Number
To multiply a fraction by a whole number, we multiply the numerator of the fraction by the whole number and denominator of the fraction by 1. Let's have a look at some examples.
Example 1. Multiply 4⁄7 by 5.
Solution.
So, the answer is 26⁄7.
Multiplication of Two Fractions
To multiply two fractions, we multiply the numerators of both the fractions and the product becomes numerator. Then, we multiply denominators of both the fractions and the product becomes denominator of the result. Let's have a look at some examples.
Example 1. Multiply 4⁄5 by 1⁄2.
Solution.
If we simplify 4⁄10 further, then it will become 2⁄5.
So, the answer is 2⁄5.
Example 2. Multiply 22⁄5 by 32⁄3.
Solution. Here, we have two mixed fractions. First, we must convert them to improper fractions, then we do the multiplication.
Improper fraction of 22⁄5 = 12⁄5
Improper fraction of 32⁄3 = 11⁄3
Now multiply
So, the answer is 812⁄15.
Please have a look at the below given video for better understanding.
Reciprocal of Fractions
Two fractions whose product is 1 are called reciprocals. For example, 2⁄3 reciprocal is 3⁄2. If the product of two fractions is equal to 1 then we can say 2⁄3 and 3⁄2 are reciprocals.
In other words, if we want to find out the reciprocal of a fraction then interchange its numerator to denominator and
denominator to numerator.
Example 1. What is the reciprocal of 5⁄7?
Solution. Reciprocal of 5⁄7 is 7⁄5.
Division of Fractions
In the division of fraction, we must find out the reciprocal of divisor and multiply it with the dividend. Let's have a look at some example.
Example 1. Divide 5⁄8 by 1⁄2.
Solution.5⁄8 ÷ 1⁄2
Here, 5⁄8 is the dividend and 1⁄2 is divisor. Reciprocal of 1⁄2 is 2⁄1.
So, the answer is 5⁄4.
Please have a look at the below given video for better understanding.
Fractions Test
Fractions Test - 1Fractions Test - 2Fractions Test - 3Class-4 Fractions Worksheet
Fractions Worksheet - 1Fractions Worksheet - 2Fractions Worksheet - 3Fractions Worksheet - 4Fractions Worksheet - 5Fractions Worksheet - 6Fractions Worksheet - 7Fractions Worksheet - 8Answer Sheet
Fractions-AnswerDownload the pdf
Copyright © 2021 LetsPlayMaths.com. All Rights Reserved.
Mixed In Key 8 5 35 5 6
Below are multiple fraction calculators capable of addition, subtraction, multiplication, division, simplification, and conversion between fractions and decimals. Fields above the solid black line represent the numerator, while fields below represent the denominator.
Mixed Numbers Calculator
Simplify Fractions Calculator
Decimal to Fraction Calculator
Fraction to Decimal Calculator
Big Number Fraction Calculator
Use this calculator if the numerators or denominators are very big integers.
In mathematics, a fraction is a number that represents a part of a whole. It consists of a numerator and a denominator. The numerator represents the number of equal parts of a whole, while the denominator is the total number of parts that make up said whole. For example, in the fraction of
| 3 |
| 8 |
| 5 |
| 8 |
Addition:
Unlike adding and subtracting integers such as 2 and 8, fractions require a common denominator to undergo these operations. One method for finding a common denominator involves multiplying the numerators and denominators of all of the fractions involved by the product of the denominators of each fraction. Multiplying all of the denominators ensures that the new denominator is certain to be a multiple of each individual denominator. The numerators also need to be multiplied by the appropriate factors to preserve the value of the fraction as a whole. This is arguably the simplest way to ensure that the fractions have a common denominator. However, in most cases, the solutions to these equations will not appear in simplified form (the provided calculator computes the simplification automatically). Below is an example using this method.
| + |
| = |
| + |
| = |
|
| EX: |
| + |
| = |
| + |
| = |
| = |
|
This process can be used for any number of fractions. Just multiply the numerators and denominators of each fraction in the problem by the product of the denominators of all the other fractions (not including its own respective denominator) in the problem.
| EX: |
| |||||||||||||||||||||||
| = |
|
An alternative method for finding a common denominator is to determine the least common multiple (LCM) for the denominators, then add or subtract the numerators as one would an integer. Using the least common multiple can be more efficient and is more likely to result in a fraction in simplified form. In the example above, the denominators were 4, 6, and 2. The least common multiple is the first shared multiple of these three numbers.
| Multiples of 2: 2, 4, 6, 8 10, 12 |
| Multiples of 4: 4, 8, 12 |
| Multiples of 6: 6, 12 |
The first multiple they all share is 12, so this is the least common multiple. To complete an addition (or subtraction) problem, multiply the numerators and denominators of each fraction in the problem by whatever value will make the denominators 12, then add the numerators.
| EX: |
| |||||||||||||||||||||||
| = |
|
Subtraction:
Fraction subtraction is essentially the same as fraction addition. A common denominator is required for the operation to occur. Refer to the addition section as well as the equations below for clarification.
| – |
| = |
| – |
| = |
|
| EX: |
| – |
| = |
| – |
| = |
| = |
|
Multiplication:
Multiplying fractions is fairly straightforward. Unlike adding and subtracting, it is not necessary to compute a common denominator in order to multiply fractions. Simply, the numerators and denominators of each fraction are multiplied, and the result forms a new numerator and denominator. If possible, the solution should be simplified. Refer to the equations below for clarification.
| × |
| = |
|
| EX: |
| × |
| = |
| = |
|
Division:
The process for dividing fractions is similar to that for multiplying fractions. In order to divide fractions, the fraction in the numerator is multiplied by the reciprocal of the fraction in the denominator. The reciprocal of a number a is simply| 1 |
| a |
Mixed In Key 8 5 35 Scale
| 3 |
| 4 |
| 4 |
| 3 |
| / |
| = |
| × |
| = |
|
| EX: |
| / |
| = |
| × |
| = |
| = |
|
Simplification:
It is often easier to work with simplified fractions. As such, fraction solutions are commonly expressed in their simplified forms.| 220 |
| 440 |
| 1 |
| 2 |
Converting between fractions and decimals:
Converting from decimals to fractions is straightforward. It does, however, require the understanding that each decimal place to the right of the decimal point represents a power of 10; the first decimal place being 101, the second 102, the third 103, and so on. Simply determine what power of 10 the decimal extends to, use that power of 10 as the denominator, enter each number to the right of the decimal point as the numerator, and simplify. For example, looking at the number 0.1234, the number 4 is in the fourth decimal place, which constitutes 104, or 10,000. This would make the fraction| 1234 |
| 10000 |
| 617 |
| 5000 |
| 1 |
| 2 |
| 5 |
| 10 |
Mixed In Key 8 5 35 C
. Knowing that the first decimal place represents 10-1,| 5 |
| 10 |
| 5 |
| 100 |
Common Engineering Fraction to Decimal Conversions
In engineering, fractions are widely used to describe the size of components such as pipes and bolts. The most common fractional and decimal equivalents are listed below.
Mixed In Key 8 5 35 D
| 64th | 32nd | 16th | 8th | 4th | 2nd | Decimal | Decimal (inch to mm) |
| 1/64 | 0.015625 | 0.396875 | |||||
| 2/64 | 1/32 | 0.03125 | 0.79375 | ||||
| 3/64 | 0.046875 | 1.190625 | |||||
| 4/64 | 2/32 | 1/16 | 0.0625 | 1.5875 | |||
| 5/64 | 0.078125 | 1.984375 | |||||
| 6/64 | 3/32 | 0.09375 | 2.38125 | ||||
| 7/64 | 0.109375 | 2.778125 | |||||
| 8/64 | 4/32 | 2/16 | 1/8 | 0.125 | 3.175 | ||
| 9/64 | 0.140625 | 3.571875 | |||||
| 10/64 | 5/32 | 0.15625 | 3.96875 | ||||
| 11/64 | 0.171875 | 4.365625 | |||||
| 12/64 | 6/32 | 3/16 | 0.1875 | 4.7625 | |||
| 13/64 | 0.203125 | 5.159375 | |||||
| 14/64 | 7/32 | 0.21875 | 5.55625 | ||||
| 15/64 | 0.234375 | 5.953125 | |||||
| 16/64 | 8/32 | 4/16 | 2/8 | 1/4 | 0.25 | 6.35 | |
| 17/64 | 0.265625 | 6.746875 | |||||
| 18/64 | 9/32 | 0.28125 | 7.14375 | ||||
| 19/64 | 0.296875 | 7.540625 | |||||
| 20/64 | 10/32 | 5/16 | 0.3125 | 7.9375 | |||
| 21/64 | 0.328125 | 8.334375 | |||||
| 22/64 | 11/32 | 0.34375 | 8.73125 | ||||
| 23/64 | 0.359375 | 9.128125 | |||||
| 24/64 | 12/32 | 6/16 | 3/8 | 0.375 | 9.525 | ||
| 25/64 | 0.390625 | 9.921875 | |||||
| 26/64 | 13/32 | 0.40625 | 10.31875 | ||||
| 27/64 | 0.421875 | 10.715625 | |||||
| 28/64 | 14/32 | 7/16 | 0.4375 | 11.1125 | |||
| 29/64 | 0.453125 | 11.509375 | |||||
| 30/64 | 15/32 | 0.46875 | 11.90625 | ||||
| 31/64 | 0.484375 | 12.303125 | |||||
| 32/64 | 16/32 | 8/16 | 4/8 | 2/4 | 1/2 | 0.5 | 12.7 |
| 33/64 | 0.515625 | 13.096875 | |||||
| 34/64 | 17/32 | 0.53125 | 13.49375 | ||||
| 35/64 | 0.546875 | 13.890625 | |||||
| 36/64 | 18/32 | 9/16 | 0.5625 | 14.2875 | |||
| 37/64 | 0.578125 | 14.684375 | |||||
| 38/64 | 19/32 | 0.59375 | 15.08125 | ||||
| 39/64 | 0.609375 | 15.478125 | |||||
| 40/64 | 20/32 | 10/16 | 5/8 | 0.625 | 15.875 | ||
| 41/64 | 0.640625 | 16.271875 | |||||
| 42/64 | 21/32 | 0.65625 | 16.66875 | ||||
| 43/64 | 0.671875 | 17.065625 | |||||
| 44/64 | 22/32 | 11/16 | 0.6875 | 17.4625 | |||
| 45/64 | 0.703125 | 17.859375 | |||||
| 46/64 | 23/32 | 0.71875 | 18.25625 | ||||
| 47/64 | 0.734375 | 18.653125 | |||||
| 48/64 | 24/32 | 12/16 | 6/8 | 3/4 | 0.75 | 19.05 | |
| 49/64 | 0.765625 | 19.446875 | |||||
| 50/64 | 25/32 | 0.78125 | 19.84375 | ||||
| 51/64 | 0.796875 | 20.240625 | |||||
| 52/64 | 26/32 | 13/16 | 0.8125 | 20.6375 | |||
| 53/64 | 0.828125 | 21.034375 | |||||
| 54/64 | 27/32 | 0.84375 | 21.43125 | ||||
| 55/64 | 0.859375 | 21.828125 | |||||
| 56/64 | 28/32 | 14/16 | 7/8 | 0.875 | 22.225 | ||
| 57/64 | 0.890625 | 22.621875 | |||||
| 58/64 | 29/32 | 0.90625 | 23.01875 | ||||
| 59/64 | 0.921875 | 23.415625 | |||||
| 60/64 | 30/32 | 15/16 | 0.9375 | 23.8125 | |||
| 61/64 | 0.953125 | 24.209375 | |||||
| 62/64 | 31/32 | 0.96875 | 24.60625 | ||||
| 63/64 | 0.984375 | 25.003125 | |||||
| 64/64 | 32/32 | 16/16 | 8/8 | 4/4 | 2/2 | 1 | 25.4 |

